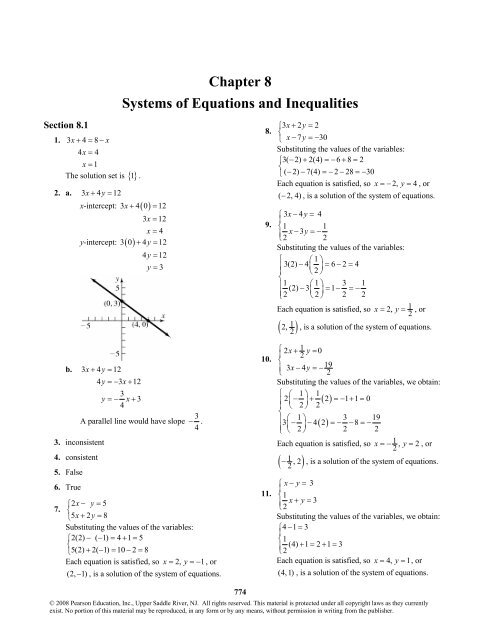
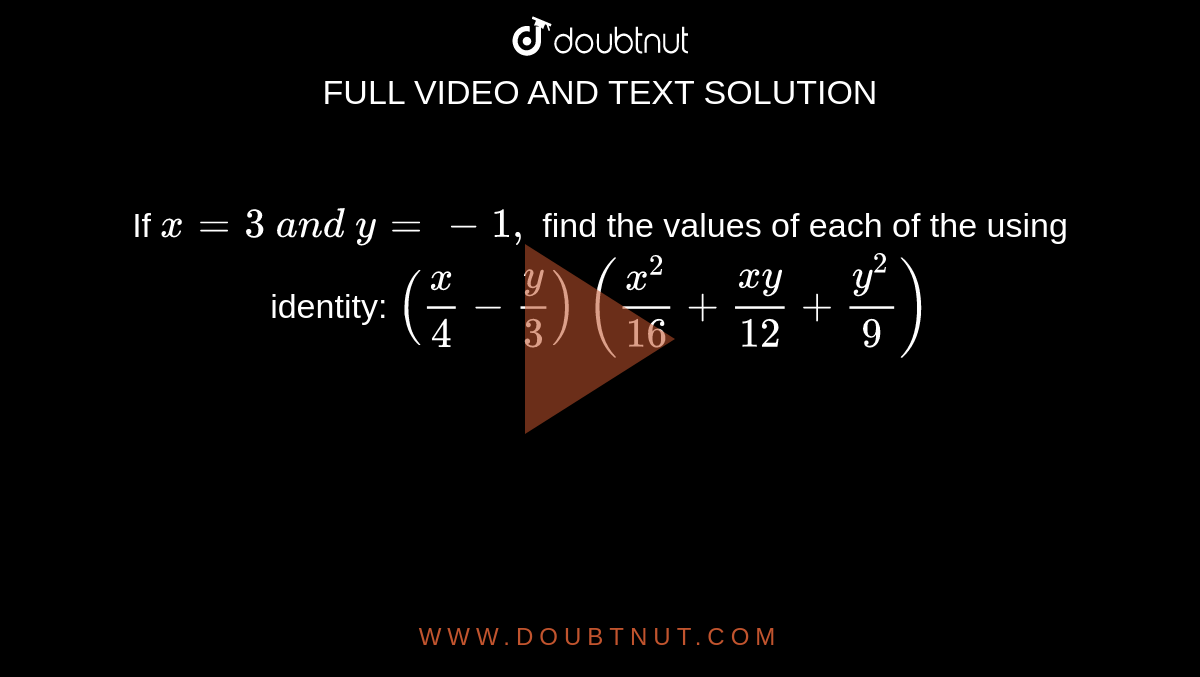
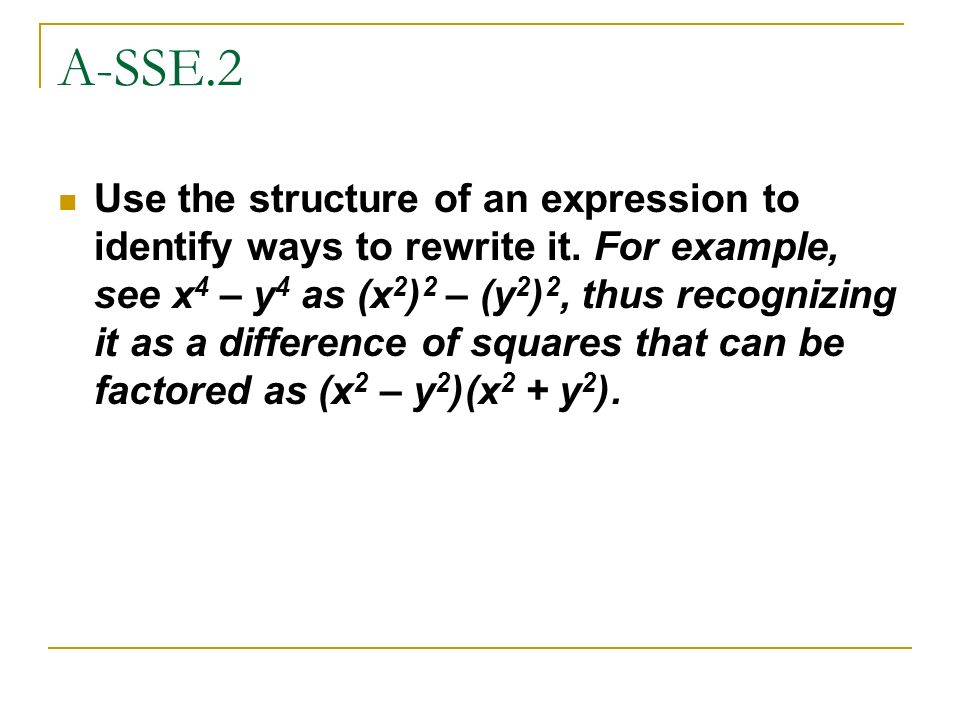
[最も共有された! √] the identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate 219128

Complete The Proof Drag And Drop The Expression To Correctly Complete The Proof Of The Polynomial Brainly Com
WebCheck that the middle term is two times the product of the numbers being squared in the first term and third term 2xy = 2 ⋅x⋅y 2 x y = 2 ⋅ x ⋅ y Rewrite the polynomial x2 2⋅x⋅yy2 xWeb An algebraic identity is an equality that holds for any values of its variables For example, the identity (xy)^2 = x^2 2xy y^2 (x y)2 = x2 2xyy2 holds for all values
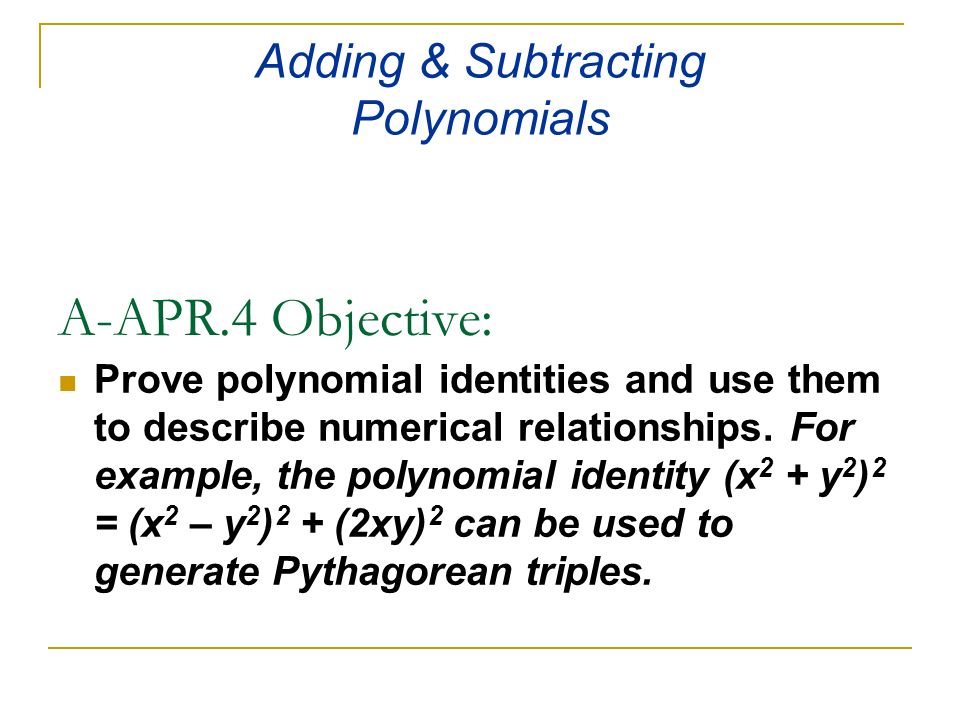
The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate
The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate-Web Use the identity (x^2y^2)^2=(x^2−y^2)^2(2xy)^2 to determine the sum of the squares of two numbers if the difference of the squares of the numbers is 5 andWeb Use the identity (x^2y^2)^2= (x^2−y^2)^2 (2xy)^2 to determine the sum of the squares of two numbers if the difference of the squares of the numbers is 5 and the

The Surface X 2 Y 2 Z 2 2xy Z A Is Shown In The Case A 0 5 Download Scientific Diagram
Web The Pythagorean triple Identity is (x 2 y 2) 2 = (x 2 y 2) 2 (2xy) 2, where c = x 2 y 2, a = x 2 y 2, and b = 2xy With x = 3 and y = 5 With x = 3 and y = 5 a = 3 2Web 1 The main integral I = ∫ x 2 − y 2 ( x 2 y 2) 2 d x can be solved using a tan substitution x = y tan θ d x = y sec 2 θ d θ So that I = ∫ y 2 ( tan 2 θ − 1) y 4 sec 4 θ y secWebAlgebra Factor x^2y^2 x2 − y2 x 2 y 2 Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 = (ab)(a−b) a 2 b 2 = ( a b) ( a b) where a
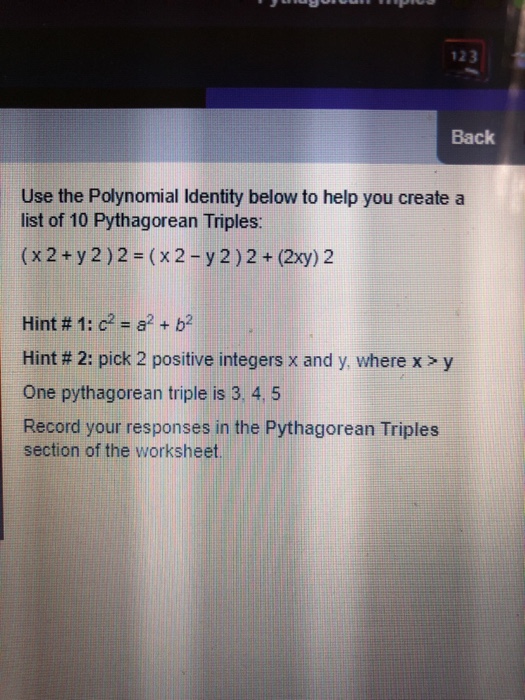
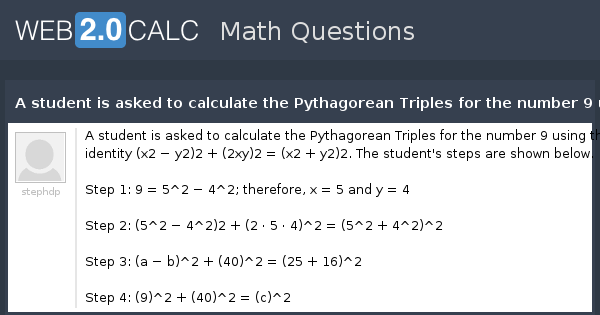
WebQuestion The identity (x^ (2)y^ (2))^ (2)= (x^ (2)y^ (2))^ (2) (2xy)^ (2) can be used to generate Pythagorean triples What Pythagorean triple could be generated using x=8 andWebProve the identity x 2y 2=(xy) 2−2xy Medium Solution Verified by Toppr x 2y 2=(xy) 2−2xy First take RHS (xy) 2−2xy We know that (xy) 2=x 22xyy 2WebYou just need to iterate through the perfect cubes smaller than 1000, and check which of them satisfies the condition (The answer is 216 ) Justification The given condition is that N ′ =
The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generateのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 |  | |
 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | 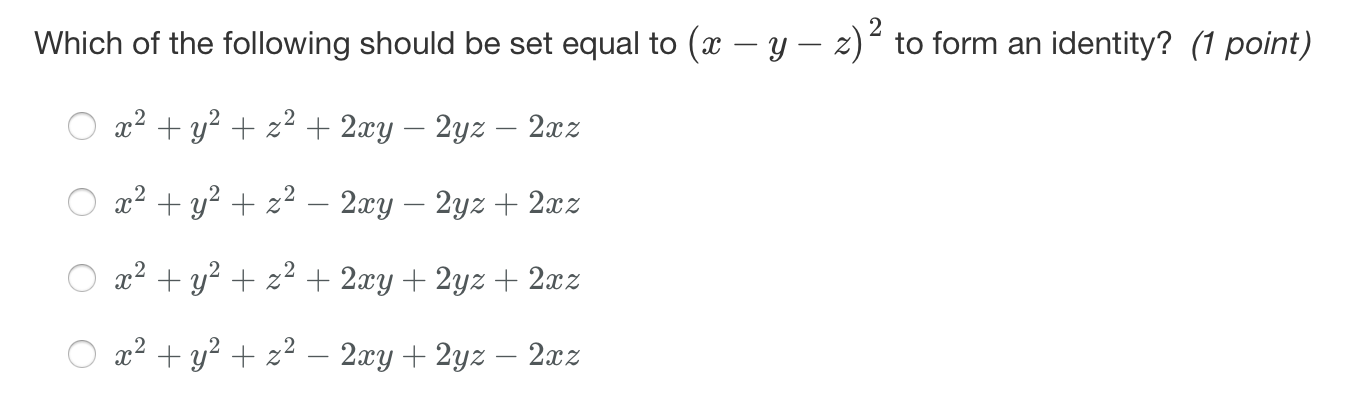 |
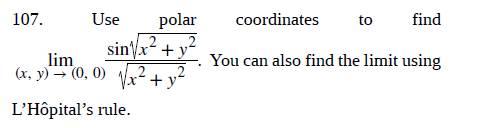 |  |  |
 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
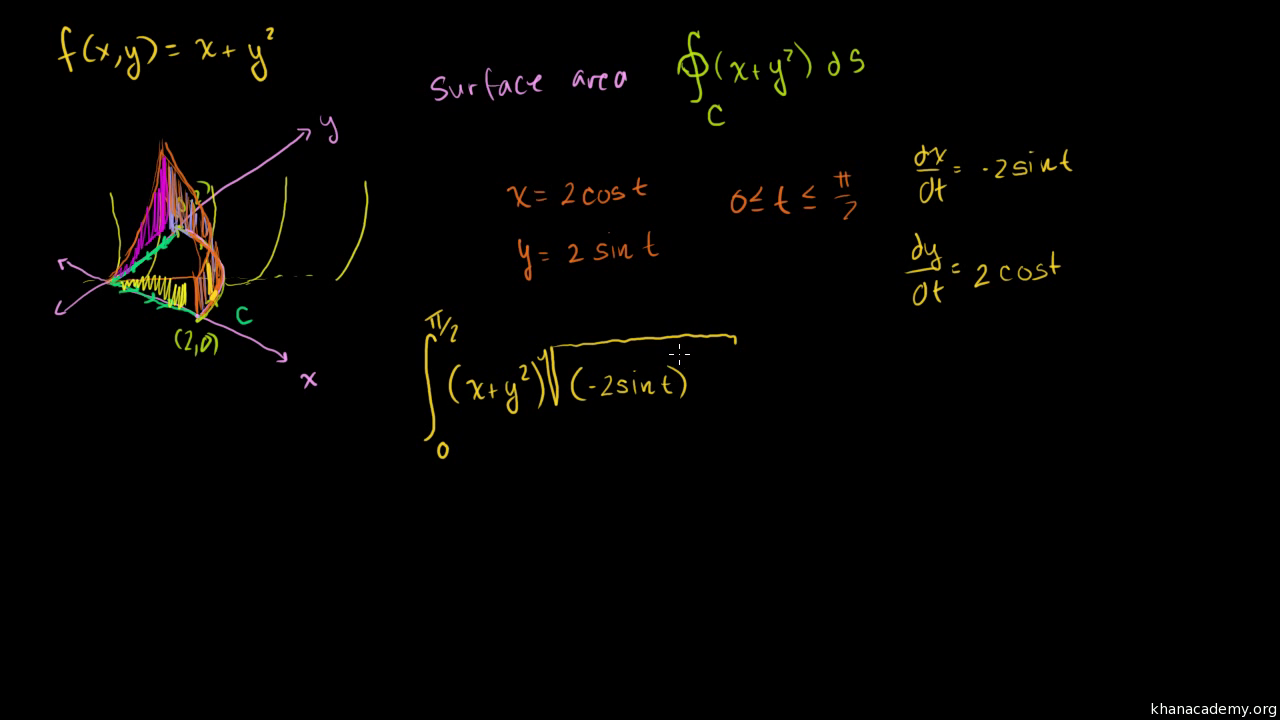 | 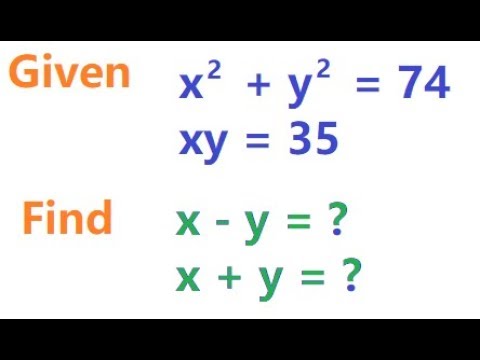 | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 | 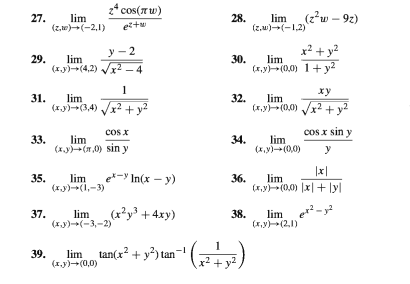 | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  |  |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
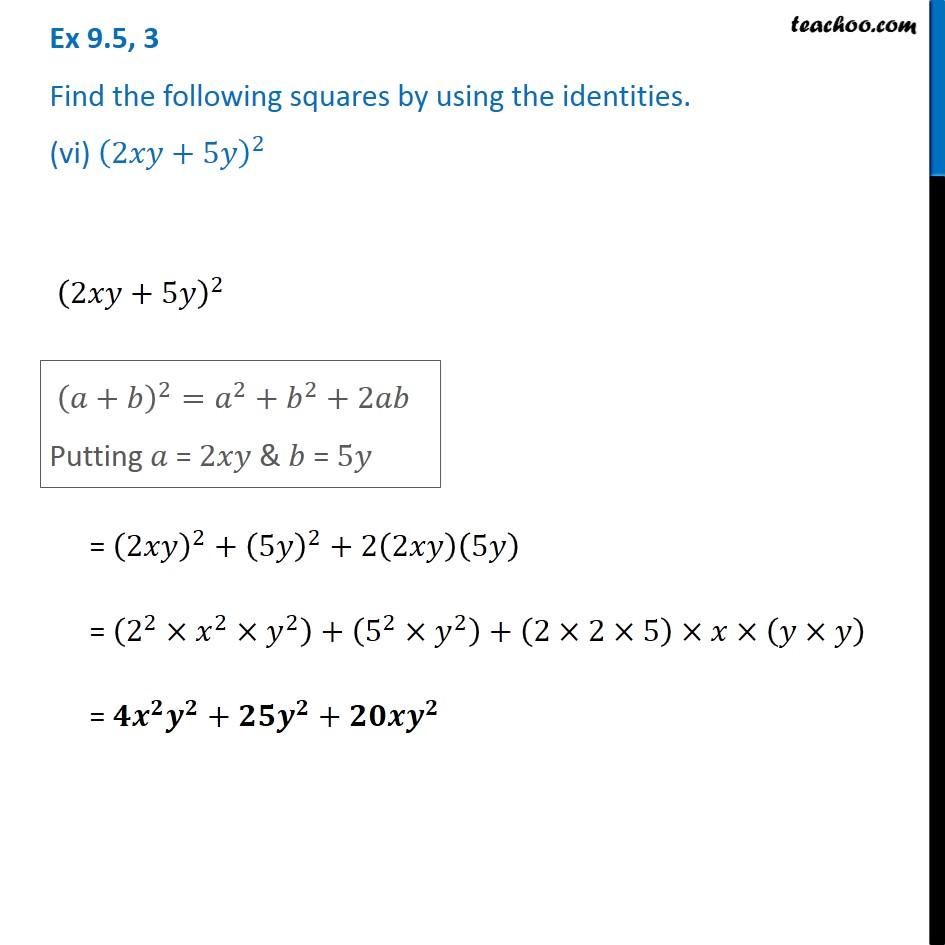 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
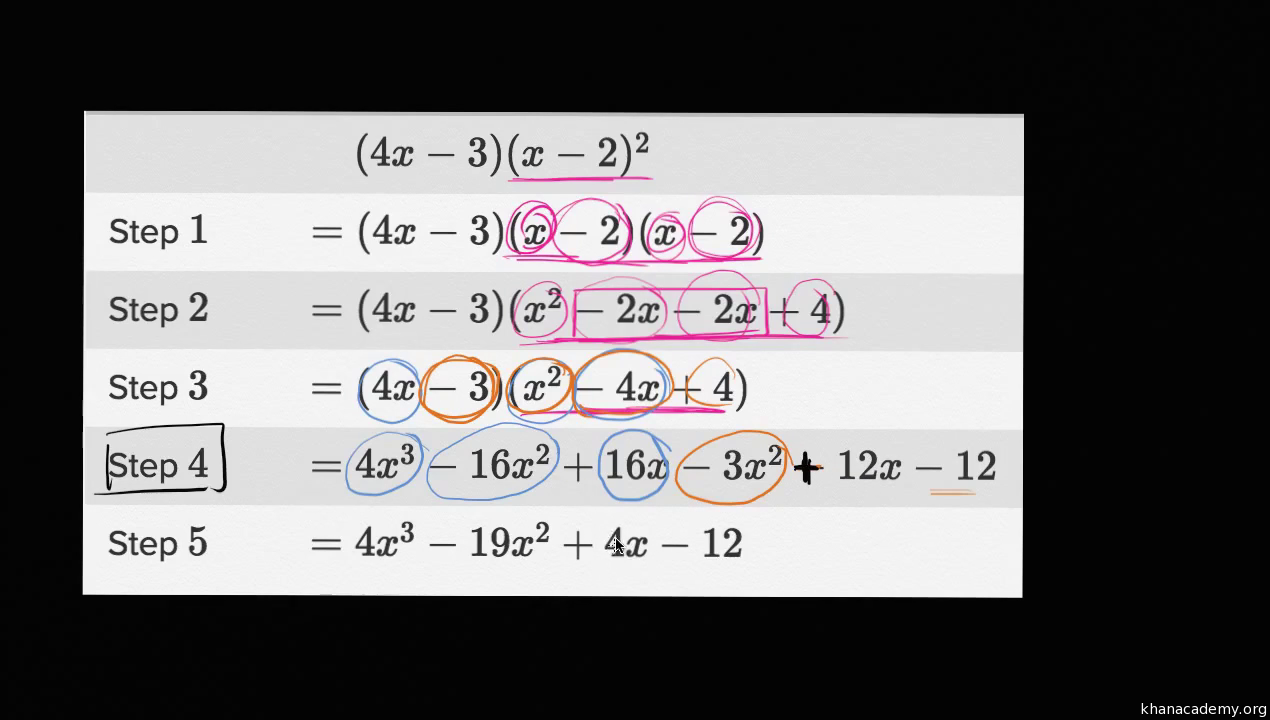 |  |  |
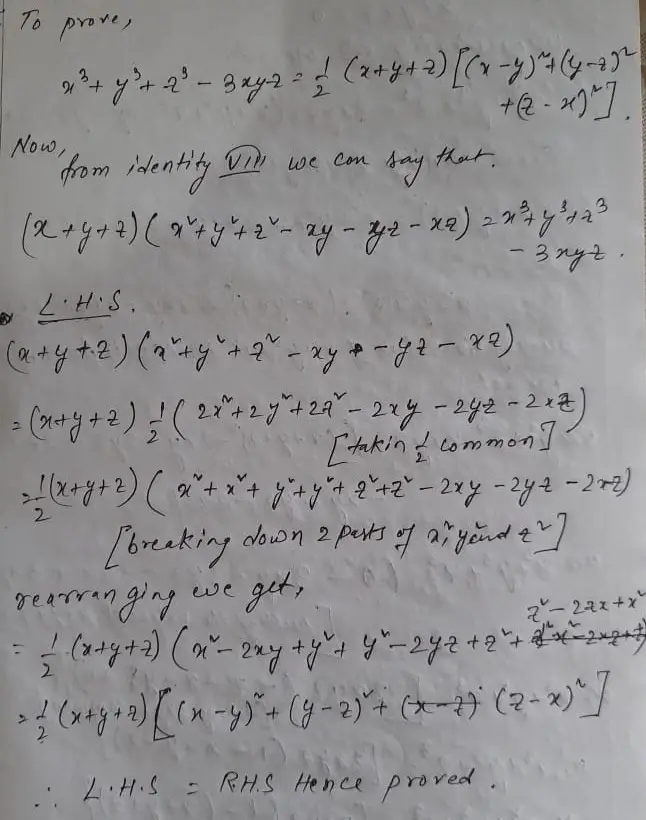 |  |  |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
「The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |
WebProduct rule, chain rule and power rule will get you the expression in terms of x,y, and y′ Gather all your y′ terms on one side, factor it out, then divide by the other factor to solveWeb The identity (x^2 y^2)^2 = (x^2 y^2)^2 (2xy)^2 can be used to generate Pythagorean triples What Pythagorean triple could be generated using x = 8 and





コメント
コメントを投稿